Question Image:
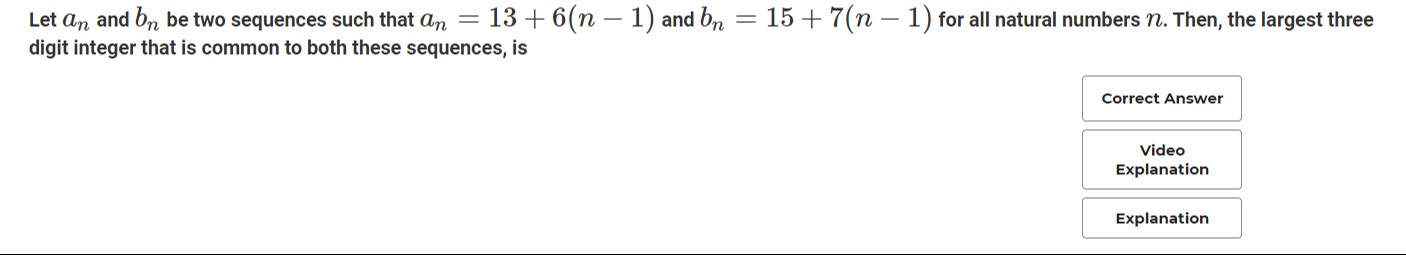
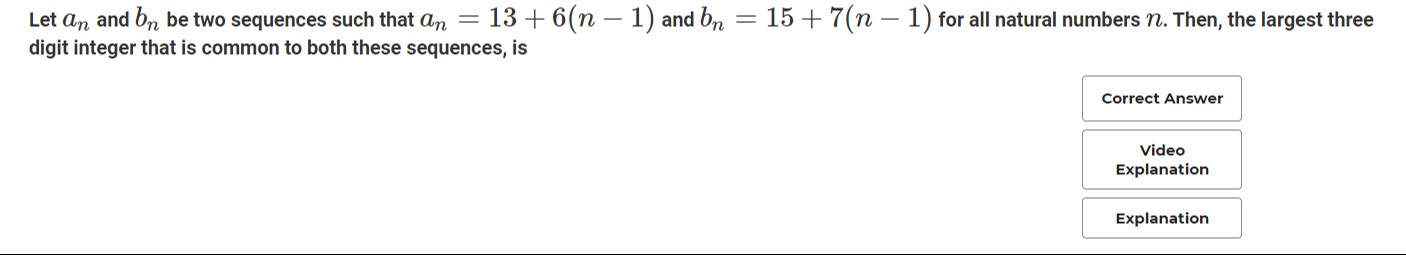
Extracted Text :
Let \( a_n \) and \( b_n \) be two sequences such that \( a_n = 13 + 6(n-1) \) and \( b_n = 15 + 7(n-1) \) for all natural numbers \( n \). Then, the largest three-digit integer that is common to both these sequences, is
Started 2 months ago by vinay kalsariya in
-
No one is replied to this thread yet. Be first to reply!
