CAT Question Paper | CAT Verbal Ability Slot
Question :
From the interior point of an equilateral triangle, perpendiculars are drawn on all three sides. The sum of the lengths of the perpendiculars is 's'. Then the area of the triangle is
Started 3 months ago by Shashank in
Option C
is the correct answer.
Explanatory Answer
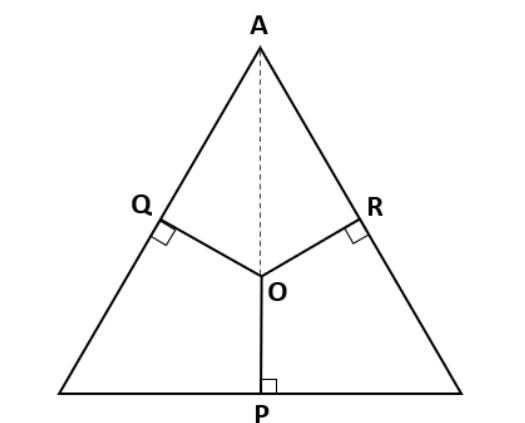
Let OP, OQ, and OR be 3 altitudes drawn from the centroid of the
equilateral triangle.
Length of all 3 p erpendicular s will be equal . Therefore, length of
OP = OQ = OR = s/3
AP is the altitude of the triangle. We know that the centroid divides
the altitude in the ratio 2:1
Hence, AO : OP = 2:1
AO = 2s/3
AP = AO + OP = s
Let side of triangle be ‘a’
Altitude AP = s = Ö 3a/2
a = 2s/ Ö 3
Area of triangle = (½)*a*s
= (½)*2s/ Ö 3*s
= s 2 / Ö 3
-
No one is replied to this question yet. Be first to reply!
