In a circle, two parallel chords on the same side of a diameter have lengths 4 cm and 6 cm. If the distance between these chords is 1 cm, then the radius of the circle, in cm, is
Started 3 months ago by Shashank in
Explanatory Answer
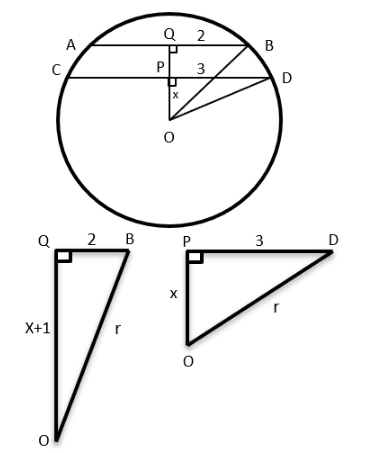
Given that Chords lie on the same side of diameter with lengths 4 cms and 6 cms
Draw a perpendicular from the origin to both the chords and mark the points of intersection as P
and Q respectively
Consider radius of circle as ‘r’ and distance OP as ‘x’
Draw lines from origin to the end of the chord and mark the points as D and B respectively
Thus, △OQB and △OPD form a right Triangle
Applying Pythagoras Theorem on both triangles,
(x+1) 2 +2 2 = r 2 ---(1)
x 2 +3 2 = r 2 ---(2)
We find that there is an increase and decrease by 1 in both equations
So, x 2 = 2 2 , x=2
r 2 = 2 2 +3 2 = 4+9 = 13
r = √13 cms
-
No one is replied to this question yet. Be first to reply!
