Live Updates
• CATKing has launched new chat bot.

• New video on Logs has been released.
13.2K
Learners
asked the doubt

Previous Year Questions
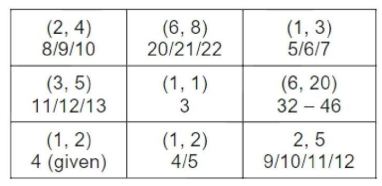
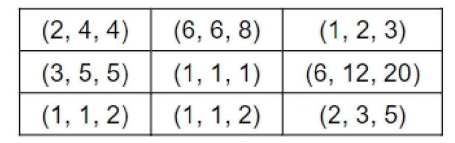
What is the total amount of money (in rupees) in the three pouches kept in the first column of the second row? [TITA]
Video Explanation

What is the maximum number of patients that the clinic can cater to on any single day?
Video Explanation

The queue is never empty on one particular Saturday. Which of the three doctors would earn the maximum amount in consultation charges on that day?
Video Explanation

On a slow Thursday, only two patients are waiting at 9 a.m. After that two patients keep arriving at exact 15 minute intervals starting at 9:15 a.m. -- i.e. at 9:15 a.m., 9:30 a.m., 9:45 a.m. etc. Then the total duration in minutes when all three doctors are simultaneously free is
Video Explanation

Three doctors
Three doctors, Dr. Ben, Dr. Kane and Dr. Wayne visit a particular clinic Monday to Saturday to see patients. Dr. Ben sees each patient for 10 minutes and charges Rs. 100/-. Dr. Kane sees each patient for 15 minutes and charges Rs. 200/-, while Dr. Wayne sees each patient for 25 minutes and charges Rs. 300/-. The clinic has three rooms numbered 1, 2 and 3 which are assigned to the three doctors as per the following table.
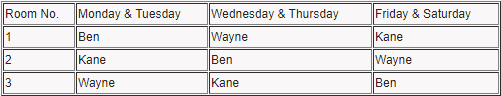
The clinic is open from 9 a.m. to 11.30 a.m. every Monday to Saturday. On arrival each patient is handed a numbered token indicating their position in the queue, starting with token number 1 every day. As soon as any doctor becomes free, the next patient in the queue enters that emptied room for consultation. If at any time, more than one room is free then the waiting patient enters the room with the smallest number. For example, if the next two patients in the queue have token numbers 7 and 8 and if rooms numbered 1 and 3 are free, then patient with token number 7 enters room number 1 and patient with token number 8 enters room number 3.
What is the maximum number of patients that the clinic can cater to on any single day?
Video Explanation

The queue is never empty on one particular Saturday. Which of the three doctors would earn the maximum amount in consultation charges on that day?
Video Explanation

On a slow Thursday, only two patients are waiting at 9 a.m. After that two patients keep arriving at exact 15 minute intervals starting at 9:15 a.m. -- i.e. at 9:15 a.m., 9:30 a.m., 9:45 a.m. etc. Then the total duration in minutes when all three doctors are simultaneously free is
Video Explanation

How many of the students surveyed supported proposal B, did not support proposal A and preferred Ragini as student union president?
Video Explanation

Students & Proposals
Students in a college are discussing two proposals --
A: a proposal by the authorities to introduce dress code on campus, and
B: a proposal by the students to allow multinational food franchises to set up outlets on college campus.
A student does not necessarily support either of the two proposals. In an upcoming election for student union president, there are two candidates in fray: Sunita and Ragini. Every student prefers one of the two candidates.
A survey was conducted among the students by picking a sample of 500 students. The following information was noted from this survey.
1) 250 students supported proposal A and 250 students supported proposal B.
2) Among the 200 students who preferred Sunita as student union president, 80% supported proposal A.
3) Among those who preferred Ragini, 30% supported proposal A.
4) 20% of those who supported proposal B preferred Sunita.
5) 40% of those who did not support proposal B preferred Ragini.
6) Every student who preferred Sunita and supported proposal B also supported proposal A.
7) Among those who preferred Ragini, 20% did not support any of the proposals.
What percentage of the students surveyed who supported both proposals A and B preferred Sunita as student union president?
Video Explanation

How many of the students surveyed supported proposal B, did not support proposal A and preferred Ragini as student union president?
Video Explanation

What percentage of the students surveyed who supported both proposals A and B preferred Sunita as student union president?
Video Explanation

What is the average rainfall of all states that have actual rainfall of 600 mm or less in 2019 and have a negative deviation from LPA?
Video Explanation

The LPA of a state for a year is defined as the average rainfall in the preceding 10 years considering the period of June-August. For example, LPA in 2018 is the average rainfall during 2009-2018 and LPA in 2019 is the average rainfall during 2010-2019. It is also observed that the actual rainfall in Gujarat in 2019 is 20% more than the rainfall in 2009. The LPA of Gujarat in 2019 is closest to
Video Explanation

Rainfall
To compare the rainfall data, India Meteorological Department (IMD) calculated the Long Period Average (LPA) of rainfall during period June-August for each of the 16 states. The figure given below shows the actual rainfall (measured in mm) during June-August, 2019 and the percentage deviations from LPA of respective states in 2018. Each state along with its actual rainfall is presented in the figure.
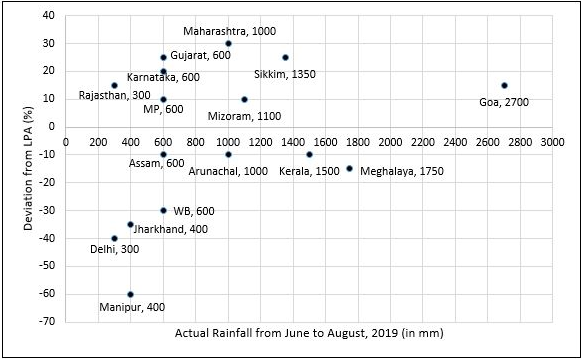
What is the average rainfall of all states that have actual rainfall of 600 mm or less in 2019 and have a negative deviation from LPA?
Video Explanation

The LPA of a state for a year is defined as the average rainfall in the preceding 10 years considering the period of June-August. For example, LPA in 2018 is the average rainfall during 2009-2018 and LPA in 2019 is the average rainfall during 2010-2019. It is also observed that the actual rainfall in Gujarat in 2019 is 20% more than the rainfall in 2009. The LPA of Gujarat in 2019 is closest to
Video Explanation

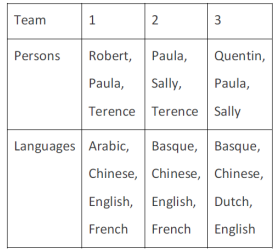
Apart from Chinese and English, which languages are spoken by Team 1?
Video Explanation

Languages spoken
In the table below the check marks indicate all languages spoken by five people: Paula, Quentin, Robert, Sally and Terence. For example, Paula speaks only Chinese and English.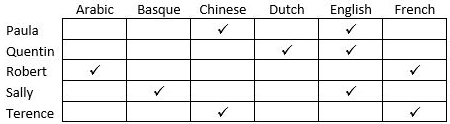
These five people form three teams, Team 1, Team 2 and Team 3. Each team has either 2 or 3 members. A team is said to speak a particular language if at least one of its members speak that language.
The following facts are known.
(1) Each team speaks exactly four languages and has the same number of members.
(2) English and Chinese are spoken by all three teams, Basque and French by exactly two teams and the other languages by exactly one team.
(3) None of the teams include both Quentin and Robert.
(4) Paula and Sally are together in exactly two teams.
(5) Robert is in Team 1 and Quentin is in Team 3.
Who among the following four is not a member of Team 2?
Video Explanation

Who among the following four people is a part of exactly two teams?
Video Explanation

Who among the five people is a member of all teams?
Video Explanation

Apart from Chinese and English, which languages are spoken by Team 1?
Video Explanation

Who among the following four is not a member of Team 2?
Video Explanation

Who among the following four people is a part of exactly two teams?
Video Explanation

Who among the five people is a member of all teams?
Video Explanation

Which of the following CANNOT be determined uniquely based on the given information?
Video Explanation

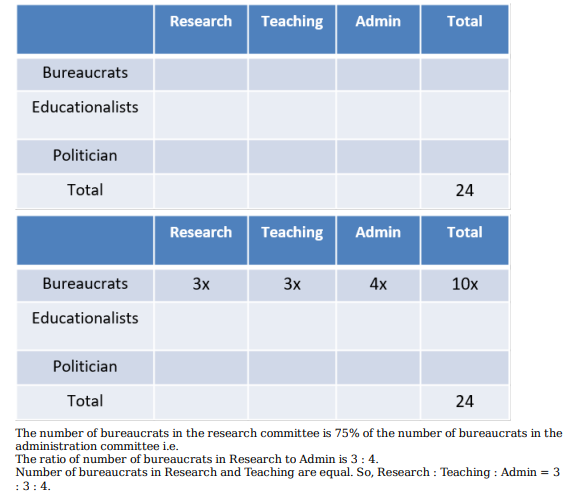
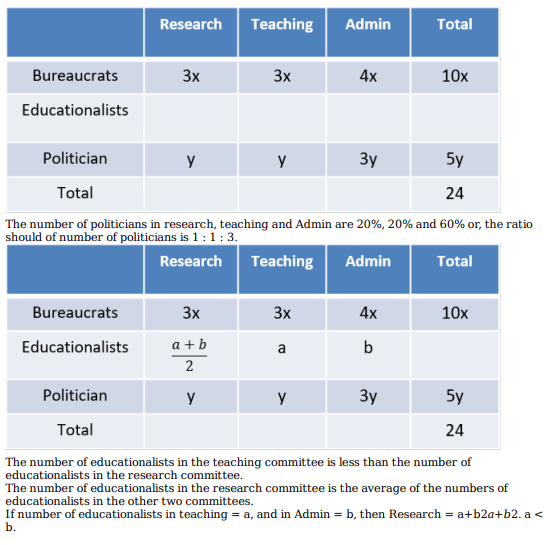
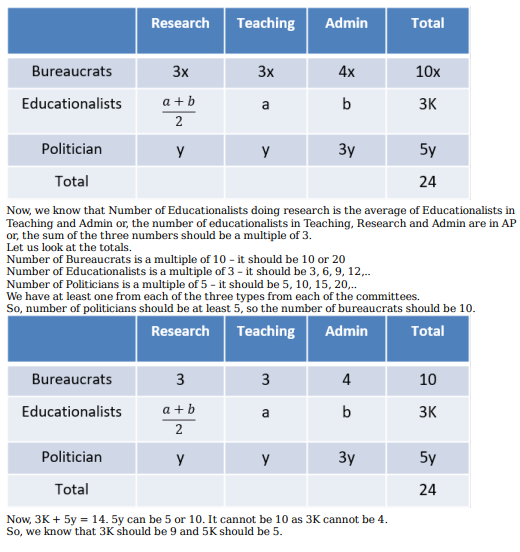
Twenty four people are part of three committees which are to look at research, teaching, and administration respectively. No two committees have any member in common. No two committees are of the same size. Each committee has three types of people: bureaucrats, educationalists, and politicians, with at least one from each of the three types in each committee. The following facts are also known about the committees:
1. The numbers of bureaucrats in the research and teaching committees are equal, while the number of bureaucrats in the research committee is 75% of the number of bureaucrats in the administration committee.
2. The number of educationalists in the teaching committee is less than the number of educationalists in the research committee. The number of educationalists in the research committee is the average of the numbers of educationalists in the other two committees.
3. 60% of the politicians are in the administration committee, and 20% are in the teaching committee.
Based on the given information, which of the following statements MUST be FALSE?
Video Explanation

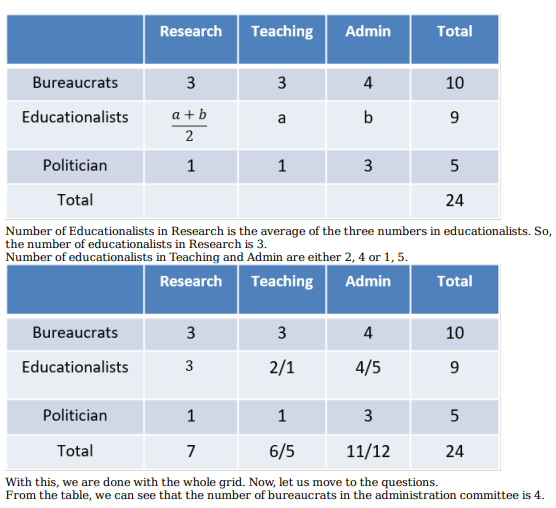
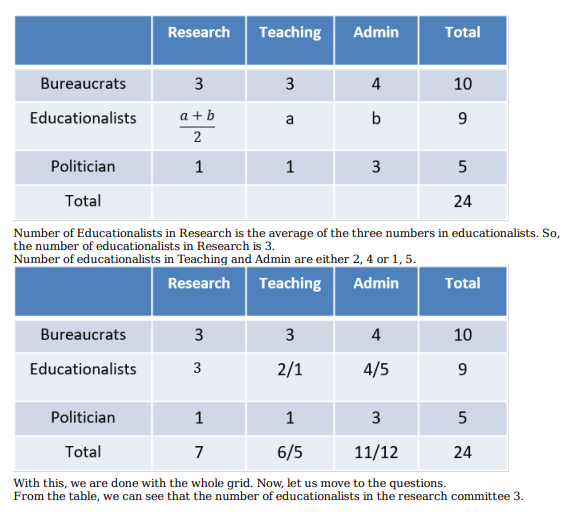
What is the number of bureaucrats in the administration committee? (TITA)
Video Explanation

What is the number of educationalists in the research committee? (TITA)
Video Explanation

Which of the following CANNOT be determined uniquely based on the given information?
Video Explanation

Based on the given information, which of the following statements MUST be FALSE?
Video Explanation

What is the number of bureaucrats in the administration committee? (TITA)
Video Explanation

What is the number of educationalists in the research committee? (TITA)
Video Explanation

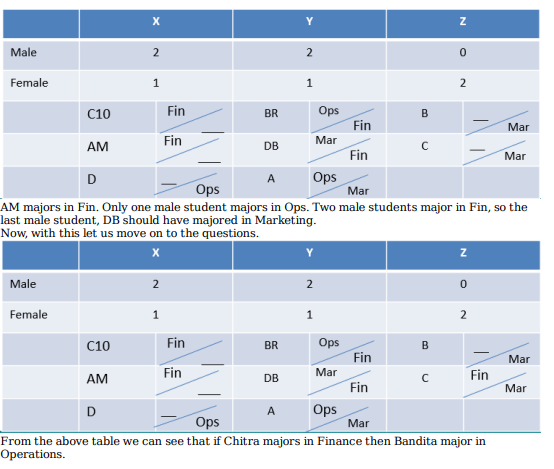
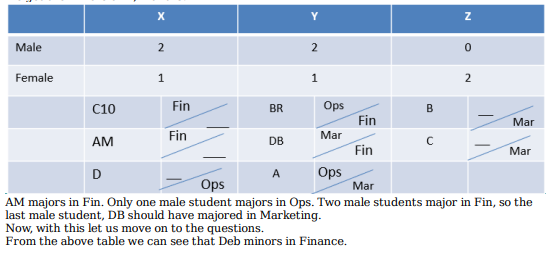
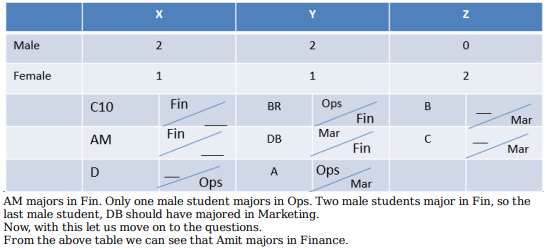
If Chitra majors in Finance, which subject does Bandita major in?
Video Explanation

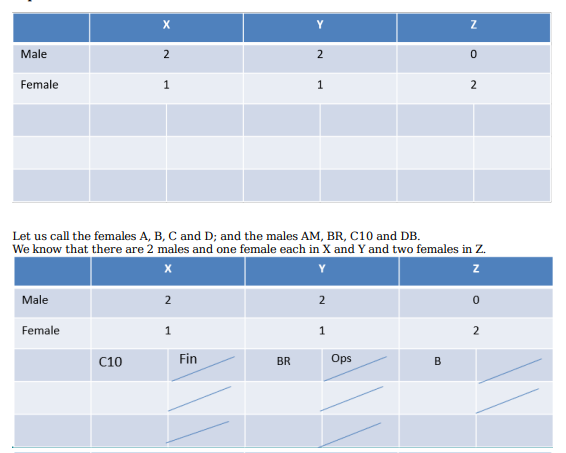
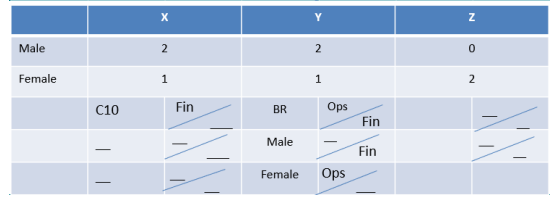
Adriana, Bandita, Chitra, and Daisy are four female students, and Amit, Barun, Chetan, and Deb are four male students. Each of them studies in one of three institutes - X, Y, and Z. Each student majors in one subject among Marketing, Operations, and Finance, and minors in a different one among these three subjects. The following facts are known about the eight students:
1. Three students are from X, three are from Y, and the remaining two students, both female, are from Z.
2. Both the male students from Y minor in Finance, while the female student from Y majors in Operations.
3. Only one male student majors in Operations, while three female students minor in Marketing.
4. One female and two male students major in Finance.
5. Adriana and Deb are from the same institute. Daisy and Amit are from the same institute.
6. Barun is from Y and majors in Operations. Chetan is from X and majors in Finance.
7. Daisy minors in Operations.
If Chitra majors in Finance, which subject does Bandita major in?
Video Explanation

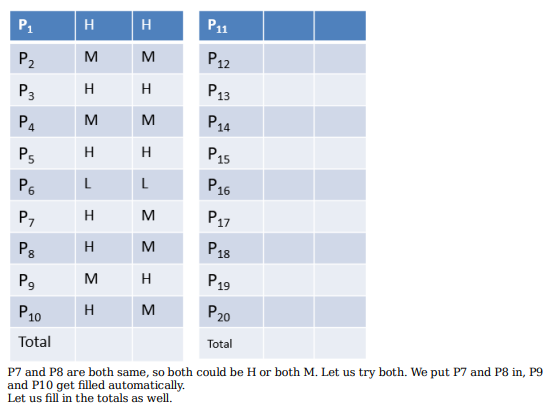
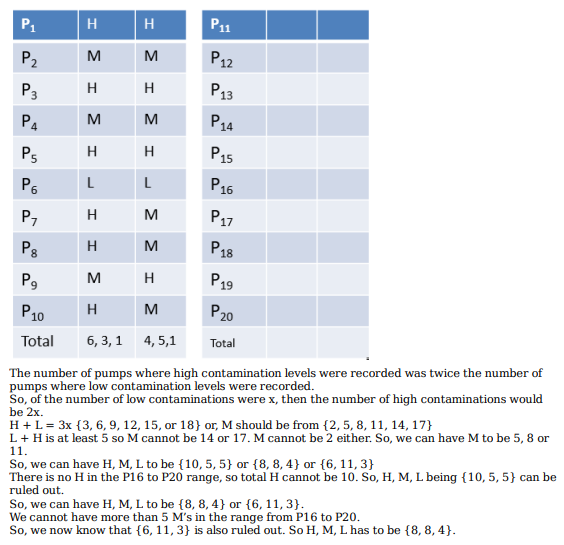
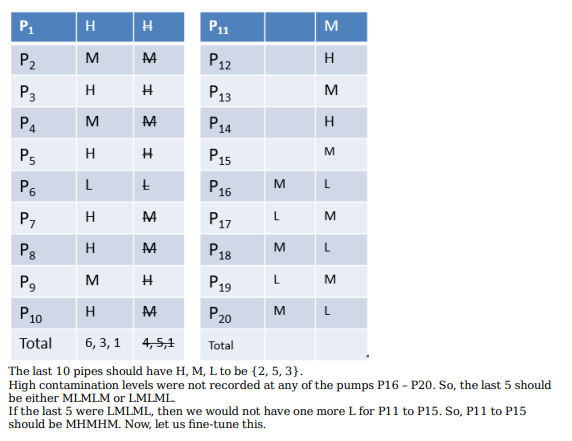
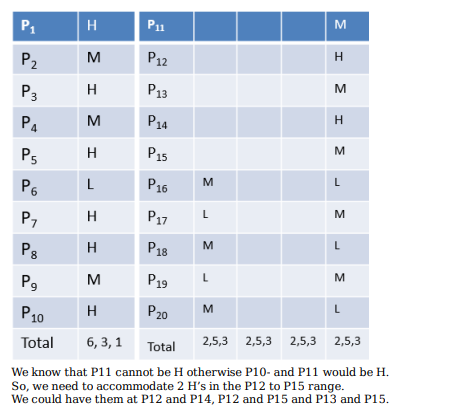
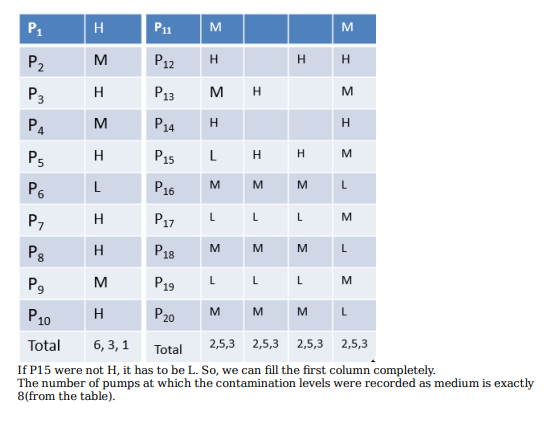
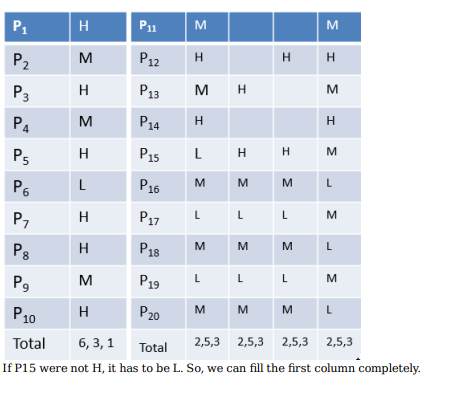
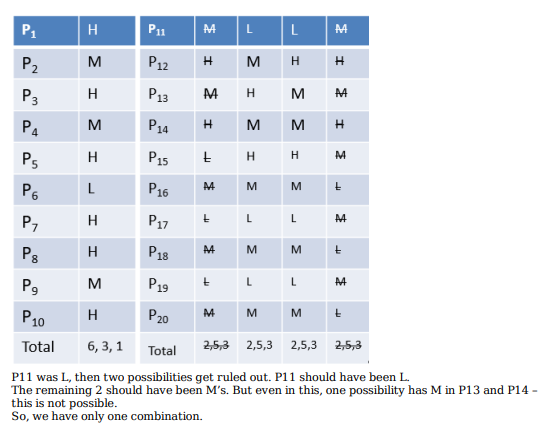
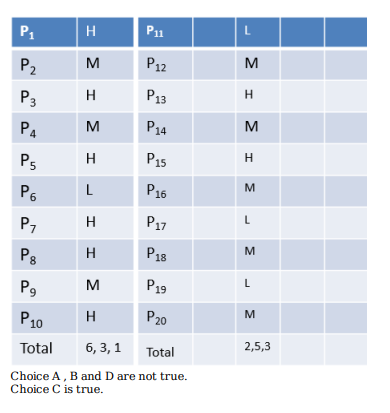
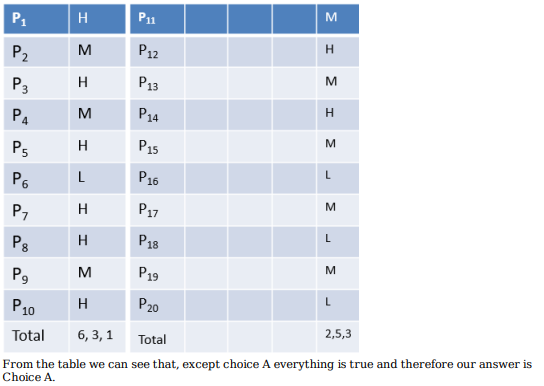
Fuel contamination levels at each of 20 petrol pumps P1, P2, …, P20 were recorded as either high, medium, or low.
1. Contamination levels at three pumps among P1 – P5 were recorded as high.
2. P6 was the only pump among P1 – P10 where the contamination level was recorded as low.
3. P7 and P8 were the only two consecutively numbered pumps where the same levels of contamination were recorded.
4. High contamination levels were not recorded at any of the pumps P16 – P20.
5. The number of pumps where high contamination levels were recorded was twice the number of pumps where low contamination levels were recorded.
What best can be said about the number of pumps at which the contamination levels were recorded as medium?
Video Explanation

If the contamination level at P11 was recorded as low, then which of the following MUST be true?
Video Explanation

If contamination level at P15 was recorded as medium, then which of the following MUST be FALSE?
Video Explanation

What best can be said about the number of pumps at which the contamination levels were recorded as medium?
Video Explanation

If the contamination level at P11 was recorded as low, then which of the following MUST be true?
Video Explanation

If contamination level at P15 was recorded as medium, then which of the following MUST be FALSE?
Video Explanation

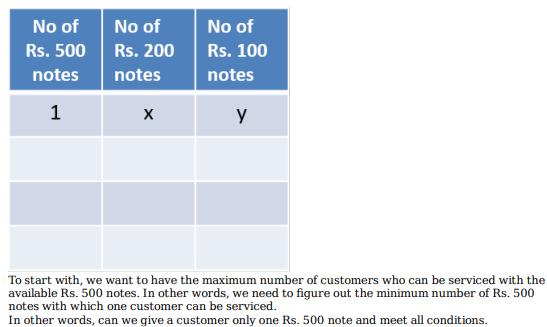
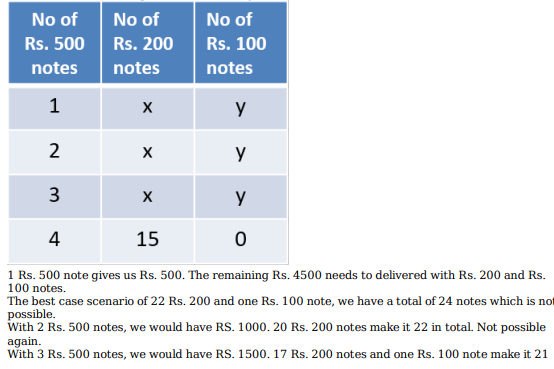
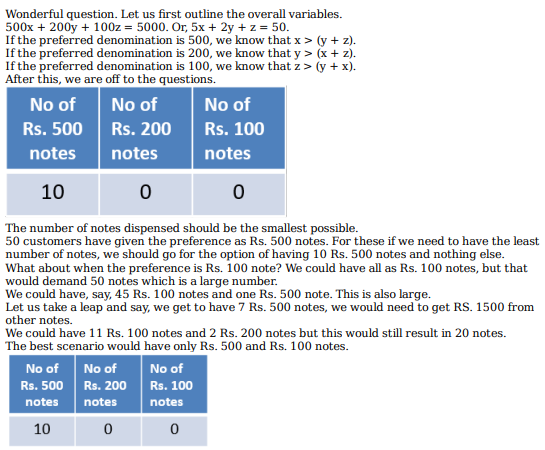
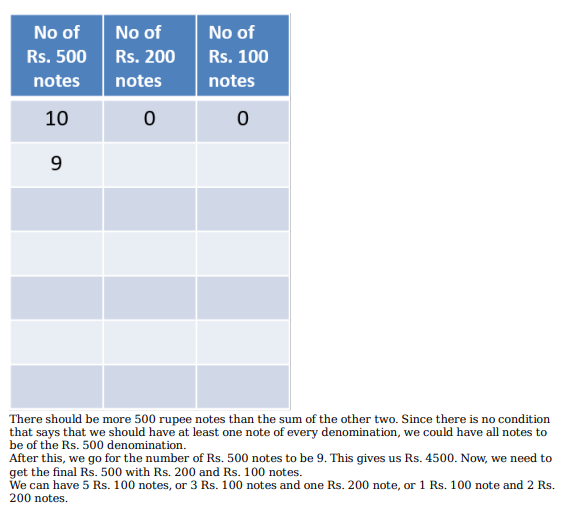
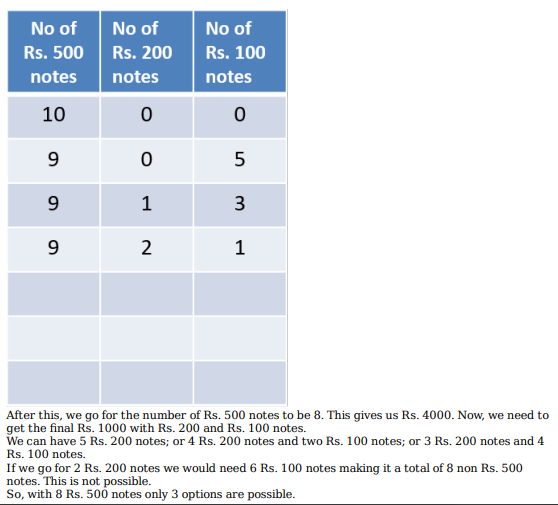
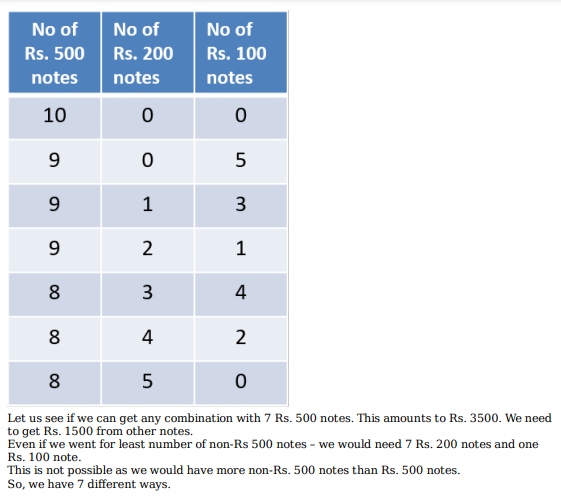
An ATM dispenses exactly Rs. 5000 per withdrawal using 100, 200 and 500 rupee notes. The ATM requires every customer to give her preference for one of the three denominations of notes. It then dispenses notes such that the number of notes of the customer’s preferred denomination exceeds the total number of notes of other denominations dispensed to her.
In how many different ways can the ATM serve a customer who gives 500 rupee notes as her preference? (TITA)
Video Explanation

In how many different ways can the ATM serve a customer who gives 500 rupee notes as her preference? (TITA)
Video Explanation

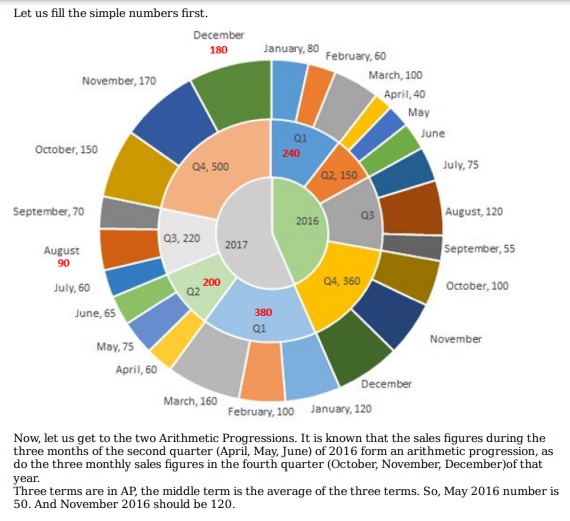
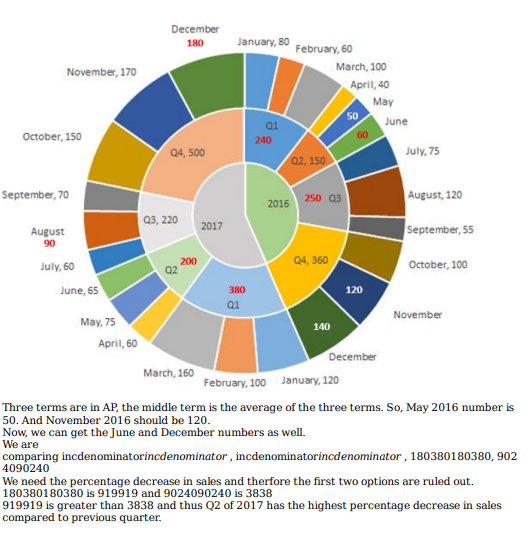
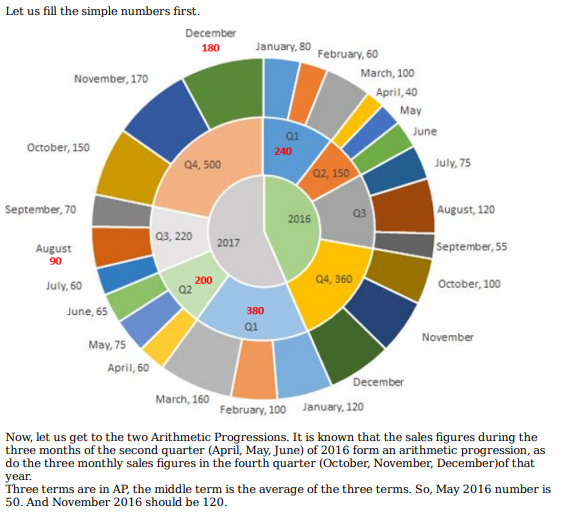
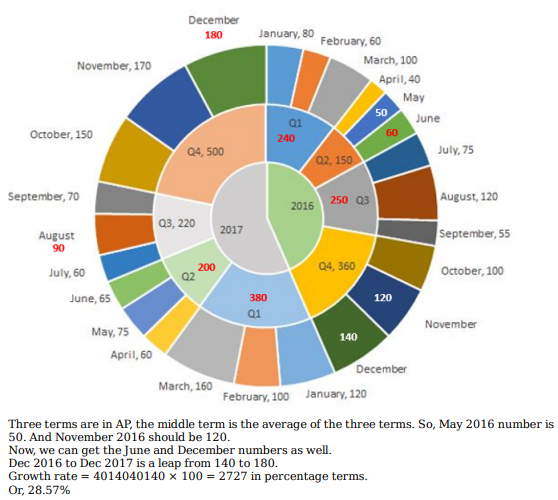
During which quarter was the percentage decrease in sales from the previous quarter’s sales the highest?
Video Explanation

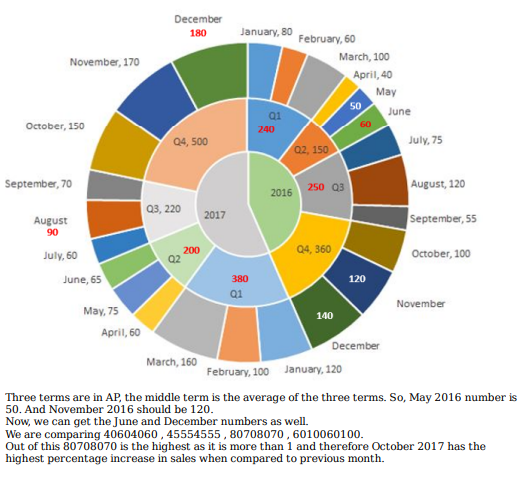
During which month was the percentage increase in sales from the previous month’s sales the highest?
Video Explanation


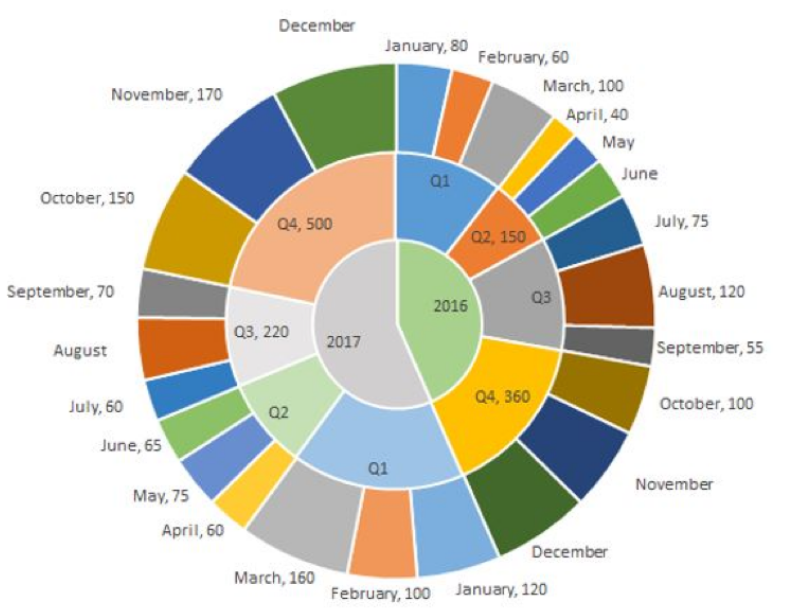
What is the percentage increase in sales in December 2017 as compared to the sales in December 2016?
Video Explanation

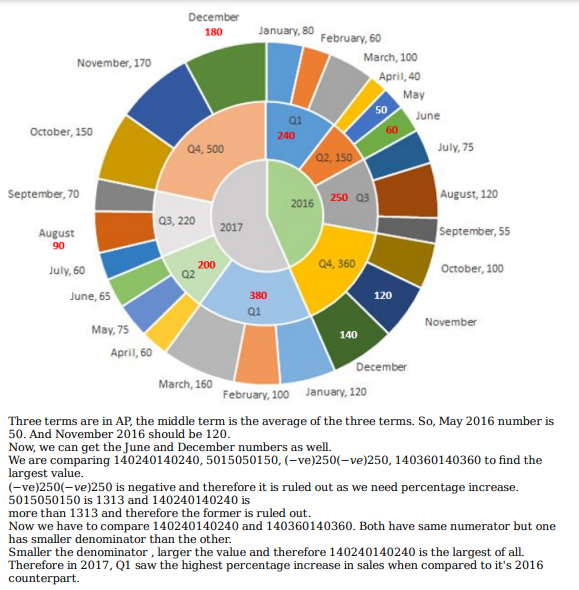
In which quarter of 2017 was the percentage increase in sales from the same quarter of 2016 the highest?
Video Explanation

During which quarter was the percentage decrease in sales from the previous quarter’s sales the highest?
Video Explanation

During which month was the percentage increase in sales from the previous month’s sales the highest?
Video Explanation

What is the percentage increase in sales in December 2017 as compared to the sales in December 2016?
Video Explanation

In which quarter of 2017 was the percentage increase in sales from the same quarter of 2016 the highest?
Video Explanation

Suppose you are allowed to make one mistake, that is, one pair of adjacent cells can have the same numeral. What is the minimum number of different numerals required to fill a 5×5 matrix?
Video Explanation

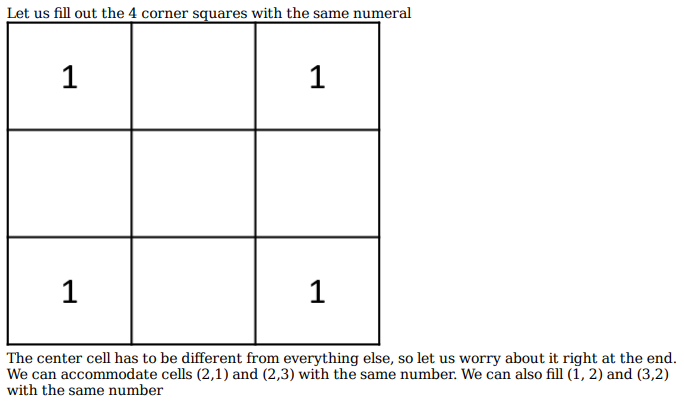
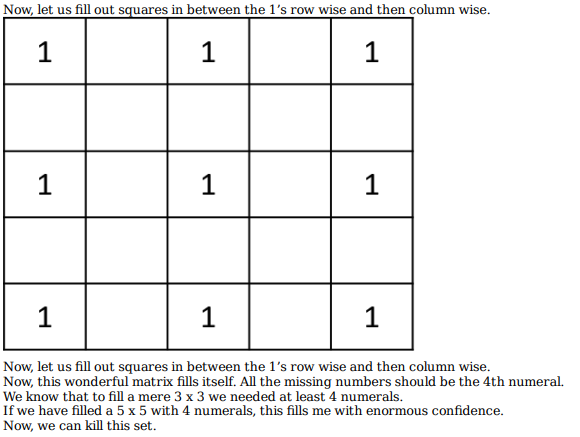
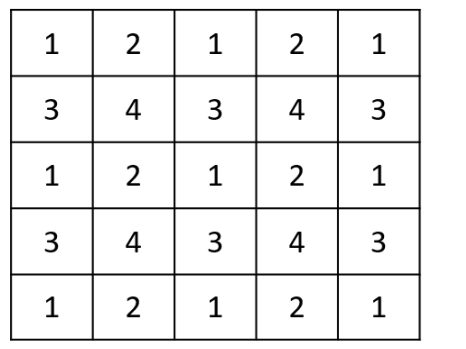
Suppose that all the cells adjacent to any particular cell must have different numerals. What is the minimum number of different numerals needed to fill a 5×5 square matrix?
Video Explanation

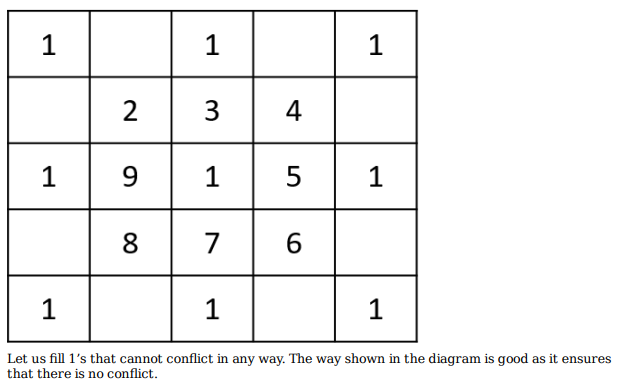
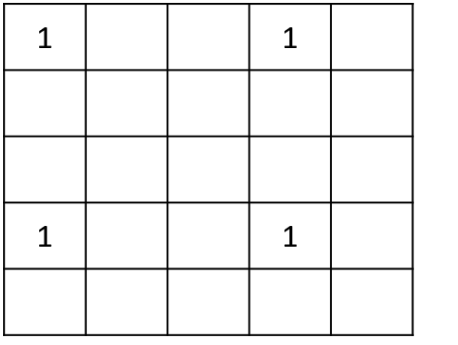
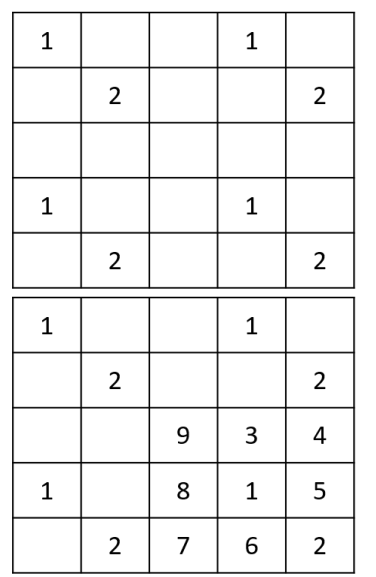
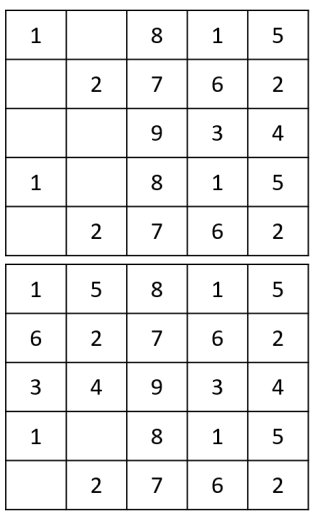
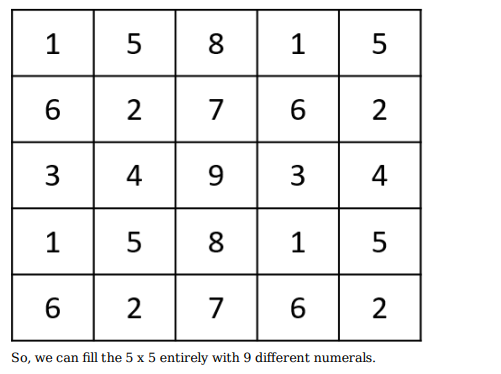
You are given an n×n square matrix to be filled with numerals so that no two adjacent cells have the same numeral. Two cells are called adjacent if they touch each other horizontally, vertically or diagonally. So a cell in one of the four corners has three cells adjacent to it, and a cell in the first or last row or column which is not in the corner has five cells adjacent to it. Any other cell has eight cells adjacent to it.
What is the minimum number of different numerals needed to fill a 3×3 square matrix? (TITA)
Video Explanation

What is the minimum number of different numerals needed to fill a 5×5 square matrix? (TITA)
Video Explanation

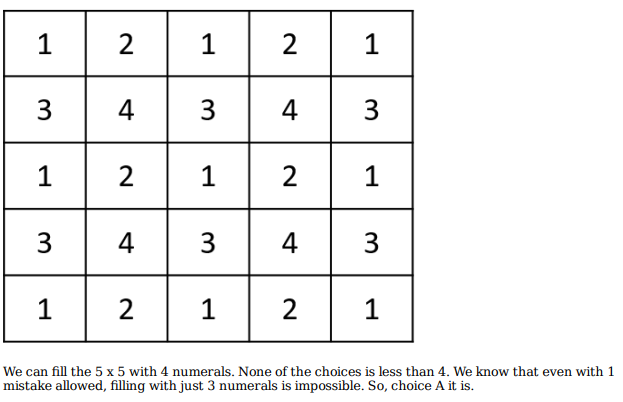
Suppose you are allowed to make one mistake, that is, one pair of adjacent cells can have the same numeral. What is the minimum number of different numerals required to fill a 5×5 matrix?
Video Explanation

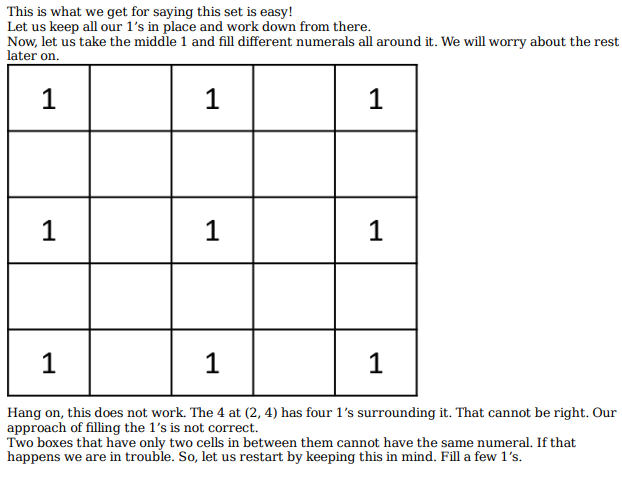
Suppose that all the cells adjacent to any particular cell must have different numerals. What is the minimum number of different numerals needed to fill a 5×5 square matrix?
Video Explanation

What is the minimum number of different numerals needed to fill a 3×3 square matrix? (TITA)
Video Explanation

Previous year papers
2023
2022
2021
2020
2019
2018