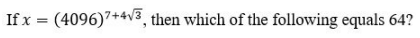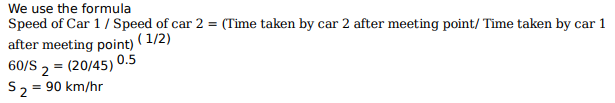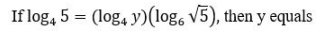Live Updates
• CATKing has launched new chat bot.
• New video on Logs has been released.
419
Learners
asked the doubt
Previous Year Questions
Video Explanation
Explanatory Answer
Area = 0.5*4*2 – 0.5*2*1 = 4-1 = 3
Video Explanation
Explanatory Answer
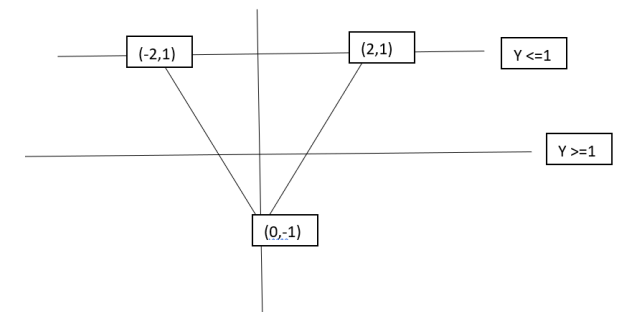
If f(5 + x) = f(5 – x) for every real x, and f (x) = 0 has four distinct real roots, then the sum of these roots is
Video Explanation
Let A, B and C be three positive integers such that the sum of A and the mean of B and C is 5. In addition, the sum of B and the mean of A and C is 7. Then the sum of A and B is
Video Explanation
Two persons are walking beside a railway track at respective speeds of 2 and 4 km per hour in the same direction. A train came from behind them and crossed them in 90 and 100 seconds, respectively. The time, in seconds, taken by the train to cross an electric post is nearest to
Video Explanation
A straight road connects points A and B. Car 1 travels from A to B and Car 2 travels from B to A, both leaving at the same time. After meeting each other, they take 45 minutes and 20 minutes, respectively, to complete their journeys. If Car 1 travels at the speed of 60 km/hr, then the speed of Car 2, in km/hr, is
Video Explanation
Explanatory Answer
Video Explanation
Explanatory Answer
Video Explanation
Explanatory Answer
On a rectangular metal sheet of area 135 sq in, a circle is painted such that the circle touches two opposite sides. If the area of the sheet left unpainted is two-thirds of the painted area then the perimeter of the rectangle in inches is:
Video Explanation
Explanatory Answer
A circle is inscribed in a rhombus with diagonals 12 cm and 16 cm. The ratio of the area of circle to the area of rhombus is:
Video Explanation
Explanatory Answer
AOC is a right-angle triangle and AC = 10
Perpendicular of a right-angle triangle = P*B/H = 8*6 / 10 = 4.8
Area of circle = P *4.8*4.8 / (0.5*12*16) = 6* P / 25
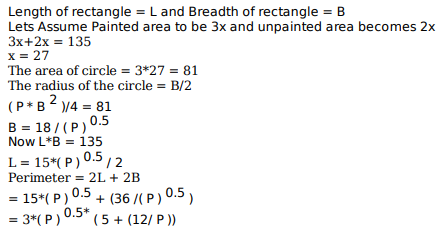
A gentleman decided to treat a few children in the following manner. He gives half of his total stock of toffees and one extra to the first child, and then the half of the remaining stock along with one extra to the second and continues giving away in this fashion. His total stock exhausts after he takes care of 5 children. How many toffees were there in his stock initially?
Video Explanation
The mean of all 4 digit even natural numbers of the form 'aabb', where a>0, is
Video Explanation
Video Explanation
Explanatory Answer
Video Explanation
Explanatory Answer
A solution, of volume 40 litres, has dye and water in the proportion 2 : 3. Water is added to the solution to change this proportion to 2 : 5. If one-fourths of this diluted solution is taken out, how many litres of dye must be added to the remaining solution to bring the proportion back to 2 : 3?
Video Explanation
Video Explanation
How many 3-digit numbers are there, for which the product of their digits is more than 2 but less than 7?
Video Explanation
Video Explanation
Explanatory Answer
Which of the following statements is FALSE?
Video Explanation
Explanatory Answer
From statement 3, Japanese and chinese did not belong to automation à A is indian
From statement 7, B and G belong to the same country and D is from Japan.
à Band G can be Indans only. Alos, H will be Japanese
From 5, E and C had some no. of papers, also E has consecutive papers i.e E wrote 2 papers
which means C also wrote 2 pages. Thus B wrote 1 paper as sum (B,C,E,F)= 6
From 3, G can belong to automation
From stat5, C and E are from different areas which can be either of manufacturing and logistics,
thus H is from Logistics
Now A cannot publish in October
H cannot publish in oct as H & A publish in same months
G cannot publish in April as B publish in April
D cannot publish in July.
Thus none of the 4 authors can publish in 4 issues, that means they publish 3 papers each.
There were exactly two papers by authors from Manufacturing area in the July issue is false.
Which of the following is the correct sequence of number of papers by authors from Automation, Manufacturing and Logistics areas, respectively?
Video Explanation
Explanatory Answer
From statement 3, Japanese and chinese did not belong to automation à A is indian
From statement 7, B and G belong to the same country and D is from Japan.
à Band G can be Indans only. Alos, H will be Japanese
From 5, E and C had some no. of papers, also E has consecutive papers i.e E wrote 2 papers
which means C also wrote 2 pages. Thus B wrote 1 paper as sum (B,C,E,F)= 6
From 3, G can belong to automation
From stat5, C and E are from different areas which can be either of manufacturing and logistics,
thus H is from Logistics
Now A cannot publish in October
H cannot publish in oct as H & A publish in same months
G cannot publish in April as B publish in April
D cannot publish in July.
Thus none of the 4 authors can publish in 4 issues, that means they publish 3 papers each.
6, 5, 7 is the correct sequence of number of papers by authors from Automation, Manufacturing
and Logistics areas, respectively.
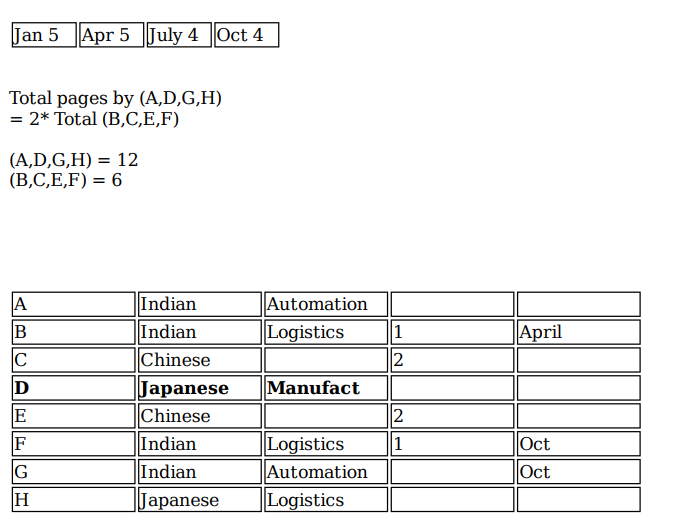
A journal plans to publish 18 research papers, written by eight authors (A, B, C, D, E, F, G, and H) in four issues of the journal scheduled in January, April, July and October. Each of the research papers was written by exactly one of the eight authors. Five papers were scheduled in each of the first two issues, while four were scheduled in each of the last two issues. Every author wrote at least one paper and at most three papers. The total number of papers written by A, D, G and H was double the total number of papers written by the other four authors. Four of the authors were from India and two each were from Japan and China. Each author belonged to exactly one of the three areas — Manufacturing, Automation and Logistics. Four of the authors were from the Logistics area and two were from the Automation area. As per the journal policy, none of the authors could have more than one paper in any issue of the journal.
The following facts are also known.
1. F, an Indian author from the Logistics area, wrote only one paper. It was scheduled in the October issue.
2. A was from the Automation area and did not have a paper scheduled in the October issue.
3. None of the Indian authors were from the Manufacturing area and none of the Japanese or Chinese authors were from the Automation area.
4. A and H were from different countries, but had their papers scheduled in exactly the same issues.
5. C and E, both Chinese authors from different areas, had the same number of papers scheduled. Further, E had papers scheduled in consecutive issues of the journal but C did not.
6. B, from the Logistics area, had a paper scheduled in the April issue of the journal.
7. B and G belonged to the same country. None of their papers were scheduled in the same issue of the journal.
8. D, a Japanese author from the Manufacturing area, did not have a paper scheduled in the July issue.
9. C and H belonged to different areas.
What is the correct sequence of number of papers written by B, C, E and G, respectively?
Video Explanation
Explanatory Answer
From statement 3, Japanese and chinese did not belong to automation à A is indian
From statement 7, B and G belong to the same country and D is from Japan.
à Band G can be Indans only. Alos, H will be Japanese
From 5, E and C had some no. of papers, also E has consecutive papers i.e E wrote 2 papers
which means C also wrote 2 pages. Thus B wrote 1 paper as sum (B,C,E,F)= 6
From 3, G can belong to automation
From stat5, C and E are from different areas which can be either of manufacturing and logistics,
thus H is from Logistics
Now A cannot publish in October
H cannot publish in oct as H & A publish in same months
G cannot publish in April as B publish in April
D cannot publish in July.
Thus none of the 4 authors can publish in 4 issues, that means they publish 3 papers each.
E publish in April and July as C cannot publish consecutive months.
1, 2, 2, 3 is the correct sequence of number of papers written by B, C, E and G, respectively
How many papers were written by Indian authors?
Video Explanation
Explanatory Answer
From statement 3, Japanese and chinese did not belong to automation à A is indian
From statement 7, B and G belong to the same country and D is from Japan.
à Band G can be Indans only. Alos, H will be Japanese
From 5, E and C had some no. of papers, also E has consecutive papers i.e E wrote 2 papers
which means C also wrote 2 pages. Thus B wrote 1 paper as sum (B,C,E,F)= 6
From 3, G can belong to automation
From stat5, C and E are from different areas which can be either of manufacturing and logistics,
thus H is from Logistics
Now A cannot publish in October
H cannot publish in oct as H & A publish in same months
G cannot publish in April as B publish in April
D cannot publish in July.
Thus none of the 4 authors can publish in 4 issues, that means they publish 3 papers each.
8 papers were written by Indian authors.
Which of the following statement(s) MUST be true?
Statement A: Every issue had at least one paper by author(s) from each country.
Statement B: Every issue had at most two papers by author(s) from each area.
Video Explanation
Explanatory Answer
From statement 3, Japanese and chinese did not belong to automation à A is indian
From statement 7, B and G belong to the same country and D is from Japan.
à Band G can be Indans only. Alos, H will be Japanese
From 5, E and C had some no. of papers, also E has consecutive papers i.e E wrote 2 papers
which means C also wrote 2 pages. Thus B wrote 1 paper as sum (B,C,E,F)= 6
From 3, G can belong to automation
From stat5, C and E are from different areas which can be either of manufacturing and logistics,
thus H is from Logistics
Now A cannot publish in October
H cannot publish in oct as H & A publish in same months
G cannot publish in April as B publish in April
D cannot publish in July.
Thus none of the 4 authors can publish in 4 issues, that means they publish 3 papers each.
Statement A: Every issue had at least one paper by author(s) from each country – Ture
Statement B: Every issue had at most two papers by author(s) from each area. – Not True
Which of the following statements is FALSE?
Video Explanation
Explanatory Answer
From statement 3, Japanese and chinese did not belong to automation à A is indian
From statement 7, B and G belong to the same country and D is from Japan.
à Band G can be Indans only. Alos, H will be Japanese
From 5, E and C had some no. of papers, also E has consecutive papers i.e E wrote 2 papers
which means C also wrote 2 pages. Thus B wrote 1 paper as sum (B,C,E,F)= 6
From 3, G can belong to automation
From stat5, C and E are from different areas which can be either of manufacturing and logistics,
thus H is from Logistics
Now A cannot publish in October
H cannot publish in oct as H & A publish in same months
G cannot publish in April as B publish in April
D cannot publish in July.
Thus none of the 4 authors can publish in 4 issues, that means they publish 3 papers each.
Every issue had exactly two papers by authors from Logistics area Is not true.
Which of the following statements is FALSE?
Video Explanation
Explanatory Answer
From statement 3, Japanese and chinese did not belong to automation à A is indian
From statement 7, B and G belong to the same country and D is from Japan.
à Band G can be Indans only. Alos, H will be Japanese
From 5, E and C had some no. of papers, also E has consecutive papers i.e E wrote 2 papers
which means C also wrote 2 pages. Thus B wrote 1 paper as sum (B,C,E,F)= 6
From 3, G can belong to automation
From stat5, C and E are from different areas which can be either of manufacturing and logistics,
thus H is from Logistics
Now A cannot publish in October
H cannot publish in oct as H & A publish in same months
G cannot publish in April as B publish in April
D cannot publish in July.
Thus none of the 4 authors can publish in 4 issues, that means they publish 3 papers each.
There were exactly two papers by authors from Manufacturing area in the July issue is false.
Which of the following is the correct sequence of number of papers by authors from Automation, Manufacturing and Logistics areas, respectively?
Video Explanation
Explanatory Answer
From statement 3, Japanese and chinese did not belong to automation à A is indian
From statement 7, B and G belong to the same country and D is from Japan.
à Band G can be Indans only. Alos, H will be Japanese
From 5, E and C had some no. of papers, also E has consecutive papers i.e E wrote 2 papers
which means C also wrote 2 pages. Thus B wrote 1 paper as sum (B,C,E,F)= 6
From 3, G can belong to automation
From stat5, C and E are from different areas which can be either of manufacturing and logistics,
thus H is from Logistics
Now A cannot publish in October
H cannot publish in oct as H & A publish in same months
G cannot publish in April as B publish in April
D cannot publish in July.
Thus none of the 4 authors can publish in 4 issues, that means they publish 3 papers each.
6, 5, 7 is the correct sequence of number of papers by authors from Automation, Manufacturing
and Logistics areas, respectively.
What is the correct sequence of number of papers written by B, C, E and G, respectively?
Video Explanation
Explanatory Answer
From statement 3, Japanese and chinese did not belong to automation à A is indian
From statement 7, B and G belong to the same country and D is from Japan.
à Band G can be Indans only. Alos, H will be Japanese
From 5, E and C had some no. of papers, also E has consecutive papers i.e E wrote 2 papers
which means C also wrote 2 pages. Thus B wrote 1 paper as sum (B,C,E,F)= 6
From 3, G can belong to automation
From stat5, C and E are from different areas which can be either of manufacturing and logistics,
thus H is from Logistics
Now A cannot publish in October
H cannot publish in oct as H & A publish in same months
G cannot publish in April as B publish in April
D cannot publish in July.
Thus none of the 4 authors can publish in 4 issues, that means they publish 3 papers each.
E publish in April and July as C cannot publish consecutive months.
1, 2, 2, 3 is the correct sequence of number of papers written by B, C, E and G, respectively
How many papers were written by Indian authors?
Video Explanation
Explanatory Answer
From statement 3, Japanese and chinese did not belong to automation à A is indian
From statement 7, B and G belong to the same country and D is from Japan.
à Band G can be Indans only. Alos, H will be Japanese
From 5, E and C had some no. of papers, also E has consecutive papers i.e E wrote 2 papers
which means C also wrote 2 pages. Thus B wrote 1 paper as sum (B,C,E,F)= 6
From 3, G can belong to automation
From stat5, C and E are from different areas which can be either of manufacturing and logistics,
thus H is from Logistics
Now A cannot publish in October
H cannot publish in oct as H & A publish in same months
G cannot publish in April as B publish in April
D cannot publish in July.
Thus none of the 4 authors can publish in 4 issues, that means they publish 3 papers each.
8 papers were written by Indian authors.
Which of the following statement(s) MUST be true?
Statement A: Every issue had at least one paper by author(s) from each country.
Statement B: Every issue had at most two papers by author(s) from each area.
Video Explanation
Explanatory Answer
From statement 3, Japanese and chinese did not belong to automation à A is indian
From statement 7, B and G belong to the same country and D is from Japan.
à Band G can be Indans only. Alos, H will be Japanese
From 5, E and C had some no. of papers, also E has consecutive papers i.e E wrote 2 papers
which means C also wrote 2 pages. Thus B wrote 1 paper as sum (B,C,E,F)= 6
From 3, G can belong to automation
From stat5, C and E are from different areas which can be either of manufacturing and logistics,
thus H is from Logistics
Now A cannot publish in October
H cannot publish in oct as H & A publish in same months
G cannot publish in April as B publish in April
D cannot publish in July.
Thus none of the 4 authors can publish in 4 issues, that means they publish 3 papers each.
Statement A: Every issue had at least one paper by author(s) from each country – Ture
Statement B: Every issue had at most two papers by author(s) from each area. – Not True
Which of the following statements is FALSE?
Video Explanation
Explanatory Answer
From statement 3, Japanese and chinese did not belong to automation à A is indian
From statement 7, B and G belong to the same country and D is from Japan.
à Band G can be Indans only. Alos, H will be Japanese
From 5, E and C had some no. of papers, also E has consecutive papers i.e E wrote 2 papers
which means C also wrote 2 pages. Thus B wrote 1 paper as sum (B,C,E,F)= 6
From 3, G can belong to automation
From stat5, C and E are from different areas which can be either of manufacturing and logistics,
thus H is from Logistics
Now A cannot publish in October
H cannot publish in oct as H & A publish in same months
G cannot publish in April as B publish in April
D cannot publish in July.
Thus none of the 4 authors can publish in 4 issues, that means they publish 3 papers each.
Every issue had exactly two papers by authors from Logistics area Is not true.
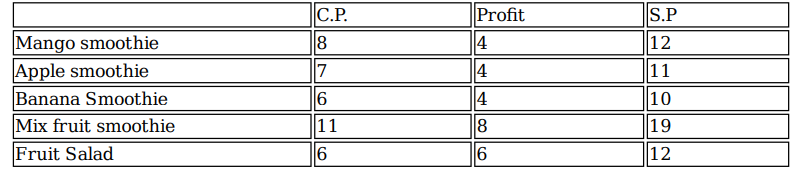
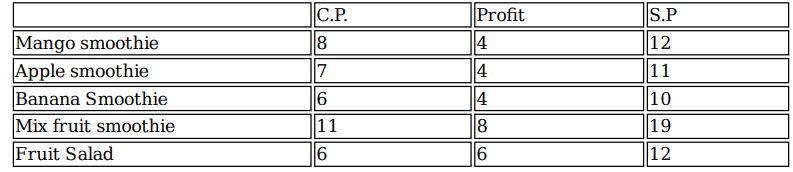
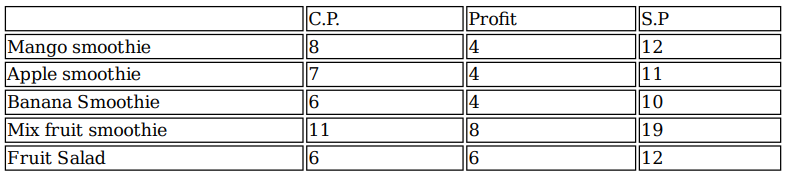
Ganga, Kaveri, and Narmada are three women who buy four raw materials (Mango, Apple, Banana and Milk) and sell five finished products (Mango smoothie, Apple smoothie, Banana smoothie, Mixed fruit smoothie and Fruit salad). Table-1 gives information about the raw materials required to produce the five finished products. One unit of a finished product requires one unit of each of the raw materials mentioned in the second column of the table.
Table-1
| Finished product | Raw materials required |
| Mango smoothie | Mango, Milk |
| Apple smoothie | Apple, Milk |
| Banana smoothie | Banana, Milk |
| Mixed fruit smoothie | Mango, Apple, Banana, Milk |
| Fruit salad | Mango, Apple, Banana |
One unit of milk, mango, apple, and banana cost ₹5, ₹3, ₹2, and ₹1 respectively. Each unit of a finished product is sold for a profit equal to two times the number of raw materials used to make that product. For example, apple smoothie is made with two raw materials (apple and milk) and will be sold for a profit of ₹4 per unit. Leftover raw materials are sold during the last business hour of the day for a loss of ₹1 per unit.
The amount, in rupees, received from sales (revenue) for each woman in each of the four business hours of the day is given in Table-2.
Table-2
| Business Hour | Ganga | Kaveri | Narmada |
| Hour 1 | 23 | 19 | 31 |
| Hour 2 | 21 | 22 | 21 |
| Hour 3 | 29 | 30 | 23 |
| Hour 4 (last hour) | 30 | 27 | 22 |
The following additional facts are known.
1. No one except possibly Ganga sold any Mango smoothie.
2. Each woman sold either zero or one unit of any single finished product in any hour.
3. Each woman had exactly one unit each of two different raw materials as leftovers.
4. No one had any banana leftover.
What BEST can be concluded about the number of units of fruit salad sold in the first hour?
Video Explanation
Explanatory Answer
GANGA
Hour 1 = 23 Mango + Apple = 12+11
Fruit salad + Apple = 12+11
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 29 Banana + MFS = 10+19
Hour 4 = 30 Mango+ Fruit S+ Leftover milk+ leftover mango
= 12+12+4+2 = 30
KAVERI
Hour 1 = 19 Mixed fruit smoothies
Hour 2 = 22 Fruit S + Banana = 10+12
Hour 3 = 29 Apple + MFS = 11+19
Hour 4 = 27 Mango+ Apple+ milk+ Banana
= 11+10+4+2 = 27
OR Banana+ fruit +milk + apple = 10+12+4+2=27
NARMADA
Hour 1 = 31 Mixed fruit smoothies + Fruit salad = 19+12
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 23 Apple + Fruit = 11+12
Hour 4 = 22 MFS + Left mango + left apple
= 19+2+1 = 22
Narmada must sell a fruit salad
Ganga may or may not sell fruit salad
è Either 1 or 2
Which of the following is NECESSARILY true?
Video Explanation
Explanatory Answer
GANGA
Hour 1 = 23 Mango + Apple = 12+11
Fruit salad + Apple = 12+11
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 29 Banana + MFS = 10+19
Hour 4 = 30 Mango+ Fruit S+ Leftover milk+ leftover mango
= 12+12+4+2 = 30
KAVERI
Hour 1 = 19 Mixed fruit smoothies
Hour 2 = 22 Fruit S + Banana = 10+12
Hour 3 = 29 Apple + MFS = 11+19
Hour 4 = 27 Mango+ Apple+ milk+ Banana
= 11+10+4+2 = 27
OR Banana+ fruit +milk + apple = 10+12+4+2=27
NARMADA
Hour 1 = 31 Mixed fruit smoothies + Fruit salad = 19+12
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 23 Apple + Fruit = 11+12
Hour 4 = 22 MFS + Left mango + left apple
= 19+2+1 = 22
Validating all the options from above data, Option C is the only option necessarily TRUE
What BEST can be concluded about the total number of units of milk the three women had in the beginning?
Video Explanation
Explanatory Answer
GANGA
Hour 1 = 23 Mango + Apple = 12+11
Fruit salad + Apple = 12+11
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 29 Banana + MFS = 10+19
Hour 4 = 30 Mango+ Fruit S+ Leftover milk+ leftover mango
= 12+12+4+2 = 30
KAVERI
Hour 1 = 19 Mixed fruit smoothies
Hour 2 = 22 Fruit S + Banana = 10+12
Hour 3 = 29 Apple + MFS = 11+19
Hour 4 = 27 Mango+ Apple+ milk+ Banana
= 11+10+4+2 = 27
OR Banana+ fruit +milk + apple = 10+12+4+2=27
NARMADA
Hour 1 = 31 Mixed fruit smoothies + Fruit salad = 19+12
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 23 Apple + Fruit = 11+12
Hour 4 = 22 MFS + Left mango + left apple
= 19+2+1 = 22
If it is known that three leftover units of mangoes were sold during the last business hour of the day, how many apple smoothies were sold during the day?
Video Explanation
Explanatory Answer
GANGA
Hour 1 = 23 Mango + Apple = 12+11
Fruit salad + Apple = 12+11
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 29 Banana + MFS = 10+19
Hour 4 = 30 Mango+ Fruit S+ Leftover milk+ leftover mango
= 12+12+4+2 = 30
KAVERI
Hour 1 = 19 Mixed fruit smoothies
Hour 2 = 22 Fruit S + Banana = 10+12
Hour 3 = 29 Apple + MFS = 11+19
Hour 4 = 27 Mango+ Apple+ milk+ Banana
= 11+10+4+2 = 27
OR Banana+ fruit +milk + apple = 10+12+4+2=27
NARMADA
Hour 1 = 31 Mixed fruit smoothies + Fruit salad = 19+12
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 23 Apple + Fruit = 11+12
Hour 4 = 22 MFS + Left mango + left apple
= 19+2+1 = 22
Ganga and Narmada already have leftover mangoes
for Kaveri we will consider Case 1 in hour 4
à 6 apple smoothies were sold
What BEST can be concluded about the number of units of fruit salad sold in the first hour?
Video Explanation
Explanatory Answer
GANGA
Hour 1 = 23 Mango + Apple = 12+11
Fruit salad + Apple = 12+11
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 29 Banana + MFS = 10+19
Hour 4 = 30 Mango+ Fruit S+ Leftover milk+ leftover mango
= 12+12+4+2 = 30
KAVERI
Hour 1 = 19 Mixed fruit smoothies
Hour 2 = 22 Fruit S + Banana = 10+12
Hour 3 = 29 Apple + MFS = 11+19
Hour 4 = 27 Mango+ Apple+ milk+ Banana
= 11+10+4+2 = 27
OR Banana+ fruit +milk + apple = 10+12+4+2=27
NARMADA
Hour 1 = 31 Mixed fruit smoothies + Fruit salad = 19+12
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 23 Apple + Fruit = 11+12
Hour 4 = 22 MFS + Left mango + left apple
= 19+2+1 = 22
Narmada must sell a fruit salad
Ganga may or may not sell fruit salad
è Either 1 or 2
Which of the following is NECESSARILY true?
Video Explanation
Explanatory Answer
GANGA
Hour 1 = 23 Mango + Apple = 12+11
Fruit salad + Apple = 12+11
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 29 Banana + MFS = 10+19
Hour 4 = 30 Mango+ Fruit S+ Leftover milk+ leftover mango
= 12+12+4+2 = 30
KAVERI
Hour 1 = 19 Mixed fruit smoothies
Hour 2 = 22 Fruit S + Banana = 10+12
Hour 3 = 29 Apple + MFS = 11+19
Hour 4 = 27 Mango+ Apple+ milk+ Banana
= 11+10+4+2 = 27
OR Banana+ fruit +milk + apple = 10+12+4+2=27
NARMADA
Hour 1 = 31 Mixed fruit smoothies + Fruit salad = 19+12
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 23 Apple + Fruit = 11+12
Hour 4 = 22 MFS + Left mango + left apple
= 19+2+1 = 22
Validating all the options from above data, Option C is the only option necessarily TRUE
What BEST can be concluded about the total number of units of milk the three women had in the beginning?
Video Explanation
Explanatory Answer
GANGA
Hour 1 = 23 Mango + Apple = 12+11
Fruit salad + Apple = 12+11
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 29 Banana + MFS = 10+19
Hour 4 = 30 Mango+ Fruit S+ Leftover milk+ leftover mango
= 12+12+4+2 = 30
KAVERI
Hour 1 = 19 Mixed fruit smoothies
Hour 2 = 22 Fruit S + Banana = 10+12
Hour 3 = 29 Apple + MFS = 11+19
Hour 4 = 27 Mango+ Apple+ milk+ Banana
= 11+10+4+2 = 27
OR Banana+ fruit +milk + apple = 10+12+4+2=27
NARMADA
Hour 1 = 31 Mixed fruit smoothies + Fruit salad = 19+12
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 23 Apple + Fruit = 11+12
Hour 4 = 22 MFS + Left mango + left apple
= 19+2+1 = 22
If it is known that three leftover units of mangoes were sold during the last business hour of the day, how many apple smoothies were sold during the day?
Video Explanation
Explanatory Answer
GANGA
Hour 1 = 23 Mango + Apple = 12+11
Fruit salad + Apple = 12+11
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 29 Banana + MFS = 10+19
Hour 4 = 30 Mango+ Fruit S+ Leftover milk+ leftover mango
= 12+12+4+2 = 30
KAVERI
Hour 1 = 19 Mixed fruit smoothies
Hour 2 = 22 Fruit S + Banana = 10+12
Hour 3 = 29 Apple + MFS = 11+19
Hour 4 = 27 Mango+ Apple+ milk+ Banana
= 11+10+4+2 = 27
OR Banana+ fruit +milk + apple = 10+12+4+2=27
NARMADA
Hour 1 = 31 Mixed fruit smoothies + Fruit salad = 19+12
Hour 2 = 21 Apple + Banana = 11+10
Hour 3 = 23 Apple + Fruit = 11+12
Hour 4 = 22 MFS + Left mango + left apple
= 19+2+1 = 22
Ganga and Narmada already have leftover mangoes
for Kaveri we will consider Case 1 in hour 4
à 6 apple smoothies were sold
Who is an acquaintance of Chandran?
Video Explanation
Explanatory Answer
Basis the information given in the question the following table can be made
Now since every person has a particular relationship with the other 6 people in the town
the sum of friends + acquaintances + strangers must be 6, thus
Now B can only have 1 friend who is also an acquaintance of A, basis the above table B can only be
friends with C or D. B can only be friends with D as C cannot be an acquaintance of A as C is a
stranger to A. Thus F & G will be strangers to A & B and vice-versa. Also, G will have D, E, F as
acquaintances and vice-versa.
D needs to have 2 strangers who will be C & F and vice-versa
Also C will be a stranger to E and vice-versa, thus F will be an acquaintance to C and vice-versa
Fani is an acquaintance of Chandran.
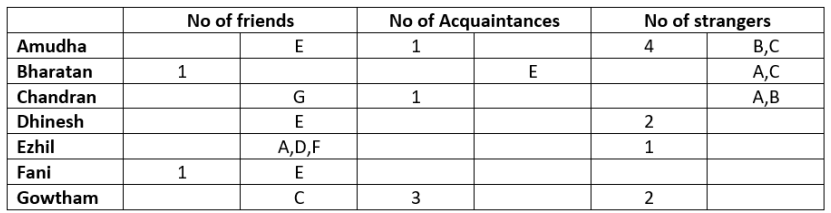
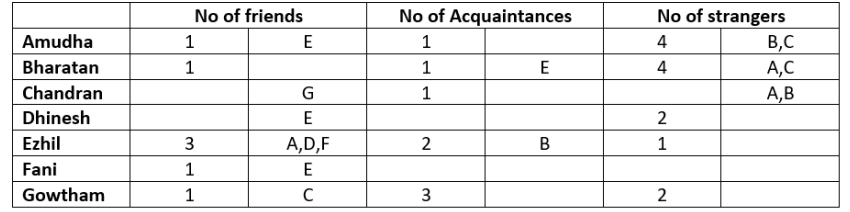
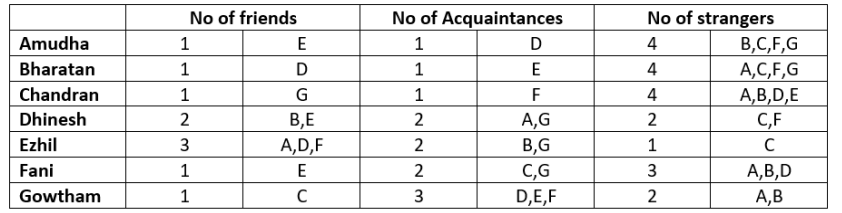
How many friends does Ezhil have?
Video Explanation
Explanatory Answer
Basis the information given in the question the following table can be made
Now since every person has a particular relationship with the other 6 people in the town
the sum of friends + acquaintances + strangers must be 6, thus
Now B can only have 1 friend who is also an acquaintance of A, basis the above table B can only be
friends with C or D. B can only be friends with D as C cannot be an acquaintance of A as C is a
stranger to A. Thus F & G will be strangers to A & B and vice-versa. Also, G will have D, E, F as
acquaintances and vice-versa.
D needs to have 2 strangers who will be C & F and vice-versa
Also C will be a stranger to E and vice-versa, thus F will be an acquaintance to C and vice-versa
Ezhil have 3 friends.
How many people are either a friend or a friend-of-a-friend of Ezhil?
Video Explanation
Explanatory Answer
Basis the information given in the question the following table can be made
Now since every person has a particular relationship with the other 6 people in the town
the sum of friends + acquaintances + strangers must be 6, thus
Now B can only have 1 friend who is also an acquaintance of A, basis the above table B can only be
friends with C or D. B can only be friends with D as C cannot be an acquaintance of A as C is a
stranger to A. Thus F & G will be strangers to A & B and vice-versa. Also, G will have D, E, F as
acquaintances and vice-versa.
D needs to have 2 strangers who will be C & F and vice-versa
Also C will be a stranger to E and vice-versa, thus F will be an acquaintance to C and vice-versa
4 people are either a friend or a friend-of-a-friend of Ezhil.
Amudha, Bharatan, Chandran, Dhinesh, Ezhil, Fani and Gowtham are seven people in a town. Any pair of them could either be strangers, acquaintances, or friends. All relationships are mutual. For example, if Amudha is a friend of Bharatan, then Bharatan is also a friend of Amudha. Similarly, if Amudha is a stranger to Bharatan, then Bharatan is also a stranger to Amudha.
Partial information about the number of friends, acquaintances, and strangers of each of these people among them is given in the table below.
| No. of Friends | No. of Acquaintances | No. of Strangers | |
| Amudha | 1 | 4 | |
| Bharatan | |||
| Chandran | 1 | ||
| Dhinesh | 2 | ||
| Ezhil | 1 | ||
| Fani | 1 | ||
| Gowtham | 3 | 2 |
The following additional facts are also known.
1. Amudha, Bharatan, and Chandran are mutual strangers.
2. Amudha, Dhinesh, and Fani are Ezil's friends.
3. Chandran and Gowtham are friends.
4. Every friend of Amudha is an acquaintance of Bharatan, and every acquaintance of Bharatan is a friend of Amudha.
5. Every friend of Bharatan is an acquaintance of Amudha, and every acquaintance of Amudha is a friend of Bharatan.
Who are Gowtham's acquaintances?
Video Explanation
Explanatory Answer
Basis the information given in the question the following table can be made
Now since every person has a particular relationship with the other 6 people in the town
the sum of friends + acquaintances + strangers must be 6, thus
Now B can only have 1 friend who is also an acquaintance of A, basis the above table B can only be
friends with C or D. B can only be friends with D as C cannot be an acquaintance of A as C is a
stranger to A. Thus F & G will be strangers to A & B and vice-versa. Also, G will have D, E, F as
acquaintances and vice-versa.
D needs to have 2 strangers who will be C & F and vice-versa
Also C will be a stranger to E and vice-versa, thus F will be an acquaintance to C and vice-versa
Dhinesh is an acquaintance of Amudha
Which of these pairs share the same type of relationship?
Video Explanation
Explanatory Answer
Basis the information given in the question the following table can be made
Now since every person has a particular relationship with the other 6 people in the town
the sum of friends + acquaintances + strangers must be 6, thus
Now B can only have 1 friend who is also an acquaintance of A, basis the above table B can only be
friends with C or D. B can only be friends with D as C cannot be an acquaintance of A as C is a
stranger to A. Thus F & G will be strangers to A & B and vice-versa. Also, G will have D, E, F as
acquaintances and vice-versa.
D needs to have 2 strangers who will be C & F and vice-versa
Also C will be a stranger to E and vice-versa, thus F will be an acquaintance to C and vice-versa
(Bharatan, Ezhil) and (Fani, Gowtham) share the same type of relationship.
Who is an acquaintance of Amudha?
Video Explanation
Explanatory Answer
Basis the information given in the question the following table can be made
Now since every person has a particular relationship with the other 6 people in the town
the sum of friends + acquaintances + strangers must be 6, thus
Now B can only have 1 friend who is also an acquaintance of A, basis the above table B can only be
friends with C or D. B can only be friends with D as C cannot be an acquaintance of A as C is a
stranger to A. Thus F & G will be strangers to A & B and vice-versa. Also, G will have D, E, F as
acquaintances and vice-versa.
D needs to have 2 strangers who will be C & F and vice-versa
Also C will be a stranger to E and vice-versa, thus F will be an acquaintance to C and vice-versa
Dhinesh is an acquaintance of Amudha
Who is an acquaintance of Chandran?
Video Explanation
Explanatory Answer
Basis the information given in the question the following table can be made
Now since every person has a particular relationship with the other 6 people in the town
the sum of friends + acquaintances + strangers must be 6, thus
Now B can only have 1 friend who is also an acquaintance of A, basis the above table B can only be
friends with C or D. B can only be friends with D as C cannot be an acquaintance of A as C is a
stranger to A. Thus F & G will be strangers to A & B and vice-versa. Also, G will have D, E, F as
acquaintances and vice-versa.
D needs to have 2 strangers who will be C & F and vice-versa
Also C will be a stranger to E and vice-versa, thus F will be an acquaintance to C and vice-versa
Fani is an acquaintance of Chandran.
How many friends does Ezhil have?
Video Explanation
Explanatory Answer
Basis the information given in the question the following table can be made
Now since every person has a particular relationship with the other 6 people in the town
the sum of friends + acquaintances + strangers must be 6, thus
Now B can only have 1 friend who is also an acquaintance of A, basis the above table B can only be
friends with C or D. B can only be friends with D as C cannot be an acquaintance of A as C is a
stranger to A. Thus F & G will be strangers to A & B and vice-versa. Also, G will have D, E, F as
acquaintances and vice-versa.
D needs to have 2 strangers who will be C & F and vice-versa
Also C will be a stranger to E and vice-versa, thus F will be an acquaintance to C and vice-versa
Ezhil have 3 friends.
How many people are either a friend or a friend-of-a-friend of Ezhil?
Video Explanation
Explanatory Answer
Basis the information given in the question the following table can be made
Now since every person has a particular relationship with the other 6 people in the town
the sum of friends + acquaintances + strangers must be 6, thus
Now B can only have 1 friend who is also an acquaintance of A, basis the above table B can only be
friends with C or D. B can only be friends with D as C cannot be an acquaintance of A as C is a
stranger to A. Thus F & G will be strangers to A & B and vice-versa. Also, G will have D, E, F as
acquaintances and vice-versa.
D needs to have 2 strangers who will be C & F and vice-versa
Also C will be a stranger to E and vice-versa, thus F will be an acquaintance to C and vice-versa
4 people are either a friend or a friend-of-a-friend of Ezhil.
Who are Gowtham's acquaintances?
Video Explanation
Explanatory Answer
Basis the information given in the question the following table can be made
Now since every person has a particular relationship with the other 6 people in the town
the sum of friends + acquaintances + strangers must be 6, thus
Now B can only have 1 friend who is also an acquaintance of A, basis the above table B can only be
friends with C or D. B can only be friends with D as C cannot be an acquaintance of A as C is a
stranger to A. Thus F & G will be strangers to A & B and vice-versa. Also, G will have D, E, F as
acquaintances and vice-versa.
D needs to have 2 strangers who will be C & F and vice-versa
Also C will be a stranger to E and vice-versa, thus F will be an acquaintance to C and vice-versa
Dhinesh is an acquaintance of Amudha
Which of these pairs share the same type of relationship?
Video Explanation
Explanatory Answer
Basis the information given in the question the following table can be made
Now since every person has a particular relationship with the other 6 people in the town
the sum of friends + acquaintances + strangers must be 6, thus
Now B can only have 1 friend who is also an acquaintance of A, basis the above table B can only be
friends with C or D. B can only be friends with D as C cannot be an acquaintance of A as C is a
stranger to A. Thus F & G will be strangers to A & B and vice-versa. Also, G will have D, E, F as
acquaintances and vice-versa.
D needs to have 2 strangers who will be C & F and vice-versa
Also C will be a stranger to E and vice-versa, thus F will be an acquaintance to C and vice-versa
(Bharatan, Ezhil) and (Fani, Gowtham) share the same type of relationship.
Who is an acquaintance of Amudha?
Video Explanation
Explanatory Answer
Basis the information given in the question the following table can be made
Now since every person has a particular relationship with the other 6 people in the town
the sum of friends + acquaintances + strangers must be 6, thus
Now B can only have 1 friend who is also an acquaintance of A, basis the above table B can only be
friends with C or D. B can only be friends with D as C cannot be an acquaintance of A as C is a
stranger to A. Thus F & G will be strangers to A & B and vice-versa. Also, G will have D, E, F as
acquaintances and vice-versa.
D needs to have 2 strangers who will be C & F and vice-versa
Also C will be a stranger to E and vice-versa, thus F will be an acquaintance to C and vice-versa
Dhinesh is an acquaintance of Amudha
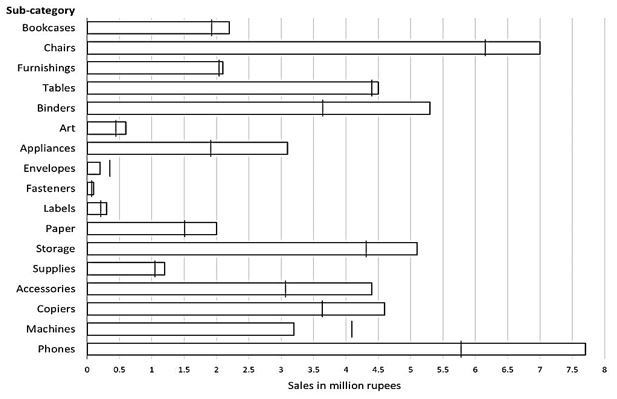
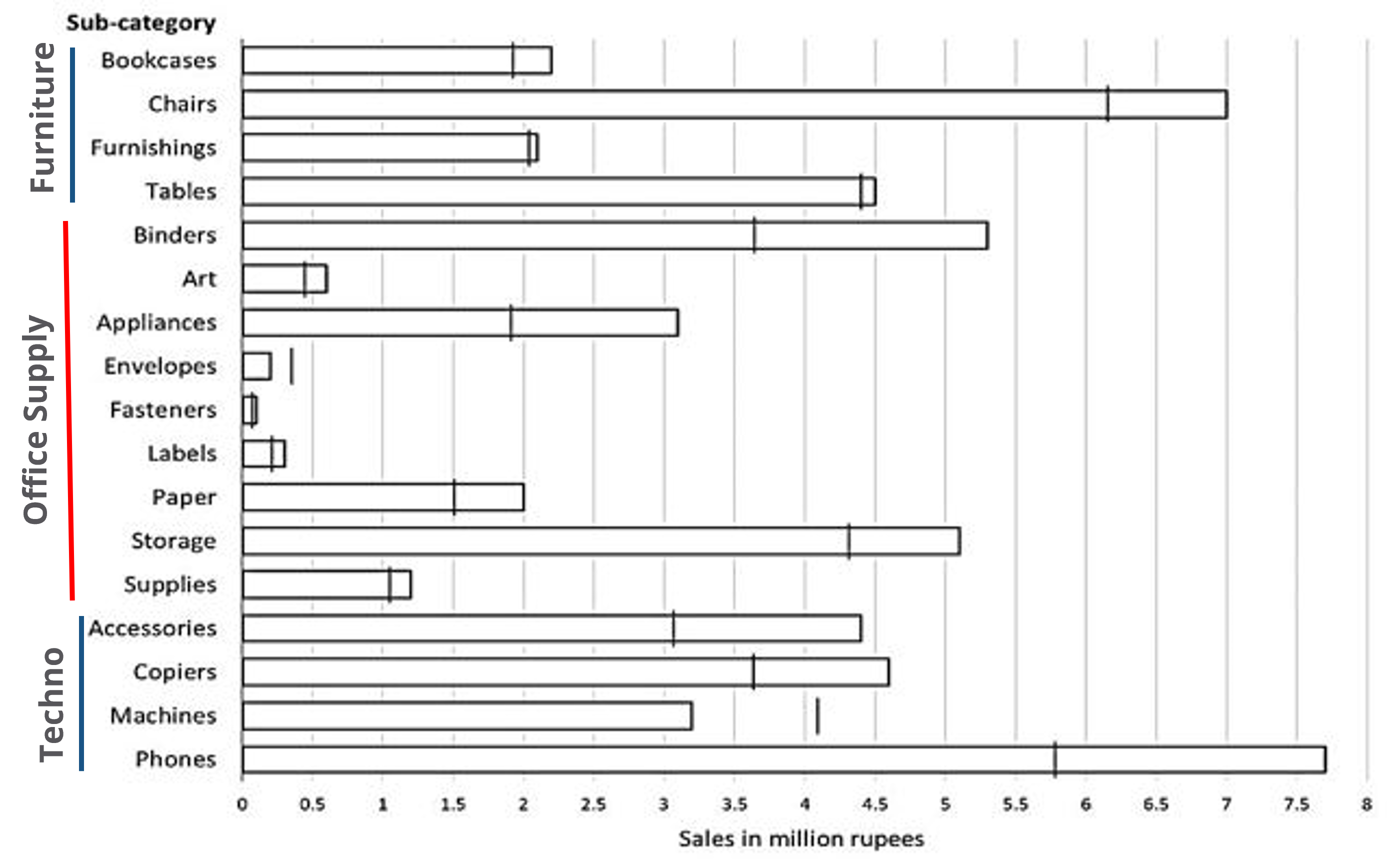
The horizontal bars in the above diagram represent 2020 aggregate sales (in ₹ million) of a company for the different subcategories of its products. The top four product subcategories (Bookcases, Chairs, Furnishings, Tables) belong to furniture product category; the bottom four product subcategories (Accessories, Copiers, Machines, Phones) belong to the technology product category while all other product subcategories belong to the office supply product category. For each of the product subcategories, there is a vertical line indicating the sales of the corresponding subcategory in 2019.
The total sales (in ₹ million) in 2019 from products in office supplies category is closest to
Video Explanation
Explanatory Answer
Binders = 3.6
Art = 0.4
Appliances = 1.9
Envelopes = 0.4
Fasteners = 0.1
Labels = 0.3
Paper = 1.5
Storage = 4.4
Supplies = 1.05
Total = 13.65
This is closest to option D
The percentage increase in sales in Furniture category from 2019 to 2020 is closest to
Video Explanation
Explanatory Answer
% increase = (15.7-14.5)/14.5 = 8%
The total sales (in ₹ million) in 2019 from products in office supplies category is closest to
Video Explanation
Explanatory Answer
Binders = 3.6
Art = 0.4
Appliances = 1.9
Envelopes = 0.4
Fasteners = 0.1
Labels = 0.3
Paper = 1.5
Storage = 4.4
Supplies = 1.05
Total = 13.65
This is closest to option D
The percentage increase in sales in Furniture category from 2019 to 2020 is closest to
Video Explanation
Explanatory Answer
% increase = (15.7-14.5)/14.5 = 8%
Each of the bottles mentioned in this question contains 50 ml of liquid. The liquid in any bottle can be 100% pure content (P) or can have certain amount of impurity (I). Visually it is not possible to distinguish between P and I. There is a testing device which detects impurity, as long as the percentage of impurity in the content tested is 10% or more.
For example, suppose bottle 1 contains only P, and bottle 2 contains 80% P and 20% I. If content from bottle 1 is tested, it will be found out that it contains only P. If content of bottle 2 is tested, the test will reveal that it contains some amount of I. If 10 ml of content from bottle 1 is mixed with 20 ml content from bottle 2, the test will show that the mixture has impurity, and hence we can conclude that at least one of the two bottles has I. However, if 10 ml of content from bottle 1 is mixed with 5 ml of content from bottle 2. the test will not detect any impurity in the resultant mixture.
